To path indicator Subpages of “Newsdetail“ To navigation To quick access To footer with other services
To content
Logo BMSD
Fakultät Architektur und Bauingenieurwesen
Studierende sitzt am Arbeitsplatz und schaut auf den Laptop. © Aliona Kardash​/​TU Dortmund

Bachelor lecture Silas Birk Blume

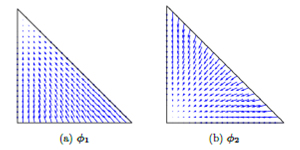
Grafik aus der Arbeit © Silas Birk Blume
Tuesday, September 28, at 3 p.m. Programming and comparison of different finite element methods: standard method, mixed FEM and least squares FEM for membrane equation

In the paper, the membrane equation is first derived and explained. Then the bilinear forms for the three methods are determined. These are then examined using the lemma of Lax-Milgram as well as the theorem of Brezzi with respect to the existence of a unique solution which can be found for all right-hand sides. This is followed by the FE formulation of the different methods using linear Lagrangian and Raviart-Thomas elements. These formulations are implemented in Python. The implementation of the mixed FEM is approached here in deviation from the scheme. Using the resulting programs, a comparison of the convergence behavior for each method with respect to the displacement variable follows.

Join Zoom Meeting:
https://tu-dortmund.zoom.us/j/94448258803?pwd=bmdrUWFwY010am5HYjIxZXJvMTFRUT09

To top of page